Here 19 cylinders intersect with axes going through opposite corners of the following polyhedra

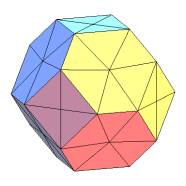
It is a truncated octahedron including the face centers. To get most isotropic oriented cylinder axes the squares being distant of 1 unit from the center of the polyhedra must have halfdiagonals 1/sqrt(3) long.

With radius 1 the surface of the intersection is

12.61582235

and its volume is a third of the surface.

The intersection has 504 facets.